AssignmentGPT Blogs
Calculus stands as a milestone in mathematics. It shapes how we think about growth, motion, and subtle changes that unfold as conditions shift. Without it, engineering would lack the precise tools needed to build reliable structures, economics would struggle to predict markets, and physics would be stuck without a language to handle continuous change. Calculus can feel daunting at first, yet it can also become an approachable companion once you pick up its core ideas. It is not some distant code reserved for academics locked in ivory towers. Instead, it lives in everyday patterns. You see it in population growth curves, stock prices, and the design of efficient machines. By examining calculus meaning and asking what is calculus definition, we learn that it is a way to break down complicated processes into manageable pieces. We will also look at different calculus types and find where they pop up in daily life.
Quick Summary
Imagine the world as a place where nothing stands still. Temperatures shift throughout the day, economies rise and fall, planets spin around suns, and sound waves travel through air. Calculus steps in as a mathematical lens to handle all that motion and variability. Without it, we might try to manage these patterns with static equations that cannot capture the flow of time. When someone asks, "what is calculus definition," the simplest reply is: it is the math of continuous change. It springs from two main pillars, derivatives and integrals. Derivatives show the rate at which something changes, and integrals show how tiny pieces add up into a bigger whole. In essence, calculus reveals a set of tools to handle complex phenomena that never sit still.
Calculus Definition
Calculus meaning often points to a framework that lets us measure how things shift as inputs vary. Traditional math might handle neat numbers or fixed shapes, but calculus grasps that reality is rarely so tidy. As values get closer to each other, as time approaches a moment, calculus uses limits to define precise concepts like instantaneous speed or exact areas under curves. Isaac Newton and Gottfried Leibniz established a robust foundation, each working separately. Their methods allowed us to approach thorny questions once considered impossible. Today, calculus stands as a bridge, connecting basic arithmetic with a grand vision of motion, growth, and accumulation. It redefines how we tackle problems, giving us a method to peel back layers of complexity. Rather than fear it, we can see it as a friend showing us hidden rules that govern dynamic systems.
How does calculus help us?
Think of fields that depend on subtle changes. Engineers design beams and supports that adapt to loads. Economists model shifting market demands. Biologists analyze how a population might increase in one season and shrink in another. Doctors interpret how a medication is absorbed and metabolized. Physicists handle gravity and acceleration, and architects shape curves in cutting-edge structures. In each scenario, calculus provides a language to measure cause and effect as values shift. By approaching problems step-by-step, by examining limits and building integrals, we can move beyond guesswork. Calculus offers a way to test ideas, refine models, and build confidence in our predictions. A single calculus problem might seem tough, but with practice, it reveals patterns hidden behind messy data.
Read More : Best Science calculator For Solving Calculus Problems 2024
A real-life application of calculus
Consider space travel. Setting a rocket’s course demands careful math. Gravity changes as altitude rises, thrust alters as fuel burns, and atmospheric drag decreases as you go higher. Calculus ties these factors together, letting scientists predict where and how fast the rocket will travel. Or think of designing a safer car braking system. By using calculus to measure speed, friction, and distances, engineers can find the best way to bring a vehicle to a smooth stop. Financial analysts apply calculus to understand how slight shifts in interest rates shape long-term investments. In medicine, doctors might use integrals to interpret signals from scans, turning what looks like random data into clear patterns. All these examples show that calculus is not a dusty theory. It is a living tool that helps us refine the world.
| Term | Description |
|---|---|
| Derivative | Measures how a function’s output changes as the input changes. |
| Differentiation | The act of finding a derivative, capturing rates of change. |
| Function | A rule that assigns each input exactly one output. |
| Integral | Summation of infinitely small increments to find totals like areas. |
| Integration | The process of determining an integral, often inverting differentiation. |
Different types of calculus
When we ask, how many calculus are there? we do not mean just counting them one by one. Instead, we recognize that calculus branches into various areas. Standard courses cover differential and integral calculus. Then come more specialized forms. Multivariable calculus handles functions of several variables, vector calculus introduces direction, and fractional calculus steps into unusual fractional powers. Differential geometry uses calculus on curved surfaces. Each branch aids in tackling fresh problems. By looking at these calculus types, we see how the field grows, meeting challenges in physics, finance, engineering, and more. There is no strict limit to how far calculus can go as our questions become more ambitious.
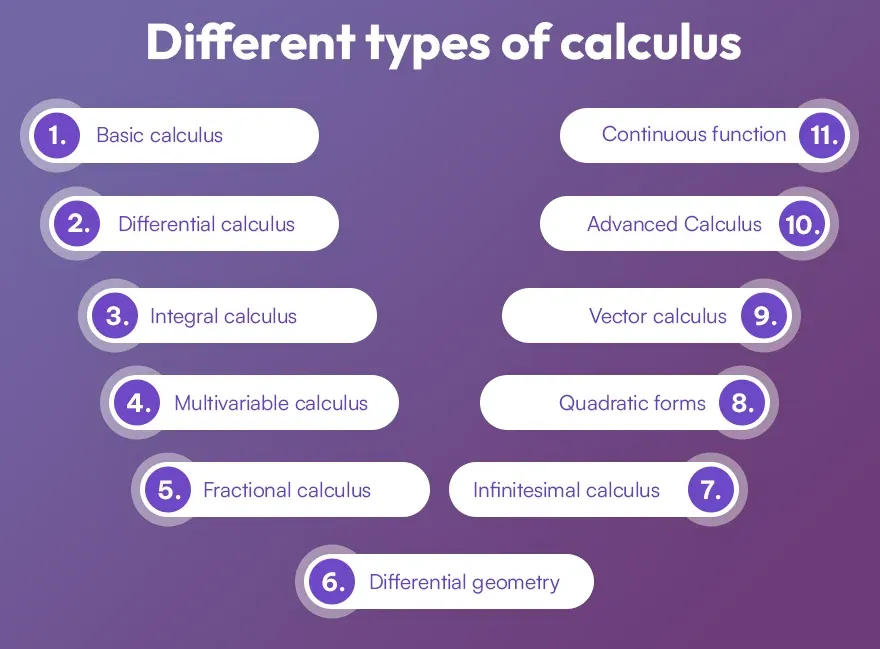
- Basic Calculus: Deals with the core concepts of derivatives and integrals.
- Differential Calculus: Focuses on calculating instantaneous rates of change.
- Integral Calculus: Concerns adding up infinitely small parts to find totals.
- Multivariable Calculus: Examines functions involving several variables simultaneously.
- Fractional Calculus: Extends differentiation and integration to fractional orders.
- Differential Geometry: Applies calculus methods to curved spaces and surfaces.
- Infinitesimal Calculus: Builds on the idea of using infinitely small increments.
- Quadratic Forms: Links calculus to algebraic forms defined by squared terms.
- Vector Calculus: Applies calculus to vector fields with direction and magnitude.
- Advanced Calculus: Involves more rigorous proofs and abstract concepts.
- Continuous Function: Focuses on functions without breaks, essential for defining derivatives.
Also read this article: 10 Best AI Tools to Solve Math Problems Instantly
Terms used in calculus
Calculus terms may sound imposing, but linking them to everyday scenarios helps. Economists rely on derivatives to forecast market changes, astronomers chart planetary movements, and musicians adjust acoustics. Game developers rely on calculus to shape dynamic, engaging worlds. Even at home, calculus informs how we manage heating, cooling, and energy use. By relating these terms to familiar domains, they transform from abstract jargon into practical tools.
| Field | Application |
|---|---|
| Economics | Forecasting profit margins, adjusting prices over time. |
| Astronomy | Plotting orbital trajectories, guiding probes through space. |
| Music | Fine-tuning acoustic designs, controlling sound waves in instruments. |
| Gaming | Enhancing realism in 3D scenes, balancing virtual environments. |
| Home Systems | Measuring wire lengths, optimizing energy use for comfort. |
Concepts important to calculus
Limits form the bedrock of calculus. They handle what happens as values approach a point, helping define instantaneous rates and areas. Derivatives rely on limits to measure sensitivity to changes, while integrals sum countless tiny pieces. Without continuity, these measures would be shaky. Continuous functions ensure that as we inch closer to a point, values do not jump unexpectedly. Infinite series take these ideas further, evaluating whether infinite sums settle into stable values. By learning these concepts, we accept these core principles and gain a way to handle complexity. Gradually, calculus becomes less of a mystery. It stands as a flexible, logical path through problems that once seemed too tangled to solve.
Conclusion
Calculus is not a lifeless collection of formulas; it reveals how change unfolds. Once you understand its patterns, you see it at work in markets, medical data, and structural designs. If you face a tough calculus problem, AssignmentGPT AI’s AI math solver can act like a reliable assistant. It breaks down complicated steps and points you in the right direction. Over time, you realize calculus is just a set of interconnected ideas that let us handle motion and accumulation with clarity. Rather than fear it, think of it as a helpful lens that sharpens your view of the world.
FAQs
1. How can I build confidence if I am new to calculus?
2. Why do derivatives and integrals get so much attention?
3. Is calculus limited to science and engineering?
4. What if I still find calculus overwhelming after some study?
5. How does technology help me learn calculus more easily?
Content writer at @AssignmentGPT
Kandarp’s world is powered by conversations, content, and creativity. With experience across branding, literature, publishing, and strategy, he has helped shape identities and stories for businesses across industries. At AssignmentGPT AI, he leads a team that blends sharp content, strong design, and local insight to turn businesses into brands that connect with people.
Master AI with
AssignmentGPT!
Get exclusive access to insider AI stories, tips and tricks. Sign up to the newsletter and be in the know!

Transform Your Studies with the Power of AssignmentGPT
Empower your academic pursuits with tools to enhance your learning speed and optimize your productivity, enabling you to excel in your studies with greater ease.
Start Your Free Trial ➤Start your success story with Assignment GPT! 🌟 Let's soar! 🚀
Step into the future of writing with our AI-powered platform. Start your free trial today and revolutionize your productivity, saving over 20 hours weekly.
Try For FREE ➤